-
Matrix Mechanics
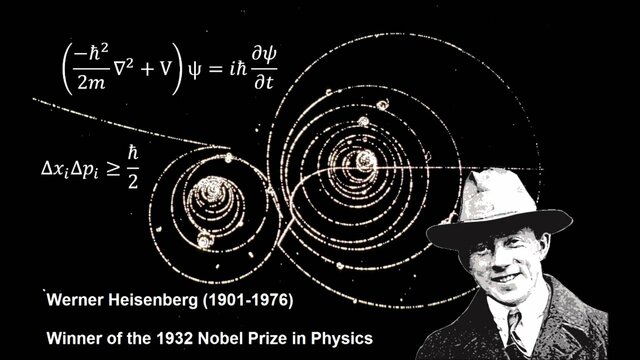
Matrix mechanics was the first complete and consistent description of quantum mechanics. This is an extension of the Bohr Model that describes how quantum leaps occur. The description is made by interpreting the physical properties of the particles as time-evolving matrices. This is the equivalent of Schrödinger wave mechanics and is the basis of Dirac notation for the wave function . -
The Uncertainty Principle
The Heisenberg uncertainty principle determines to what extent we can obtain measurement accuracy. The uncertainty principle does not result from imperfections of the measuring devices or the measurement itself. It results from the nature of things, from the dual nature of particles. This is the limit of knowledge that cannot be crossed. -
Nobel Price
In 1932 he was awarded the Nobel Prize in Physics for developing the theoretical foundations of quantum mechanics, the use of which allowed, to discover two allotropic forms of hydrogen (the so-called triplet and normal). He received the award in Stockholm in 1933. -
Unified Field Theory
In the postwar period Heisenberg continued his search for a comprehensive quantum field theory, utilizing the “scattering matrix” approach and returning to the notion of a minimum universal length as a key feature. In 1958 he proposed a unified field theory which he saw as a symmetry-based approach to the proliferation of particles then under way. -
